Y intercept of parabola

First, identify the slope and a point on the graph. Using a table or a graph, identify two points shown. Using this information, find the rise and run to identify the slope. Start by calculating the rise and run to find the slope.

The graph of a quadratic function is a U-shaped curve called a parabola. One important feature of the graph is that it has an extreme point, called the vertex. If the parabola opens up, the vertex represents the lowest point on the graph, or the minimum value of the quadratic function. If the parabola opens down, the vertex represents the highest point on the graph, or the maximum value. In either case, the vertex is a turning point on the graph. The graph is also symmetric with a vertical line drawn through the vertex, called the axis of symmetry. Determine the vertex, axis of symmetry, zeros, and y -intercept of the parabola shown below.

Y intercept of parabola

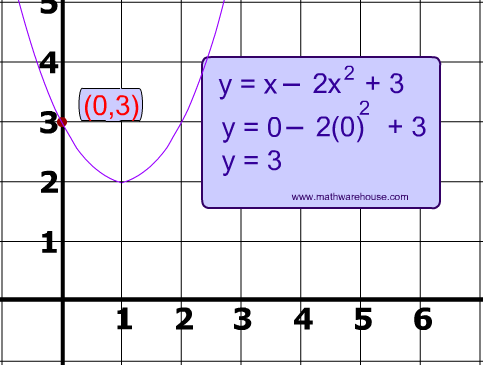
A parabola is a visual representation of a quadratic function. Each parabola contains a y-intercept, the point at which the function crosses the y-axis. Learn the tools you need to find the y-intercept using the graph of a quadratic function and the equation of a quadratic function. Finding the y-intercept of a parabola can be tricky. Although the y-intercept is hidden, it does exist. Use the equation of the function to find the y- intercept. The y-intercept has two parts: the x-value and the y-value. Note that the x-value is always zero. So, plug in zero for x and solve for y:. The y -intercept is 0, The y -intercept is 0,0. Use limited data to select advertising. Create profiles for personalised advertising.

In either case, the vertex is a turning point on the graph. Understanding the X-Intercept of a Quadratic Function.

The following graphs are two typical parabolas their x-intercepts are marked by red dots, their y-intercepts are marked by a pink dot, and the vertex of each parabola is marked by a green dot:. We say that the first parabola opens upwards is a U shape and the second parabola opens downwards is an upside down U shape. In order to graph a parabola we need to find its intercepts, vertex, and which way it opens. Notice that the x -intercepts of any graph are points on the x -axis and therefore have y -coordinate 0. If the equation factors we can find the points easily, but we may have to use the quadratic formula in some cases. If the solutions are imaginary, that means that the parabola has no x -intercepts is strictly above or below the x -axis and never crosses it. If the solutions are real, but irrational radicals then we need to approximate their values and plot them.

The y-intercept is the point where the graph intersects the y-axis. There are two types of intercepts that a function can have. They are the x-intercept and the y-intercept. An intercept of a function is a point where the graph of the function cuts the axis. The y intercept of a graph is the point where the graph intersects the y-axis. We know that the x-coordinate of any point on the y-axis is 0. So the x-coordinate of a y-intercept is 0. Here is an example of a y-intercept.

Y intercept of parabola

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Intro to parabolas. About About this video Transcript. Graphs of quadratic functions all have the same shape which we call "parabola. For example, they are all symmetric about a line that passes through their vertex. This video covers this and other basic facts about parabolas.

Hatsune miku contact lenses

Example: Identifying the Characteristics of a Parabola Determine the vertex, axis of symmetry, zeros, and y -intercept of the parabola shown below. The y -intercept of any graph is a point on the y -axis and therefore has x -coordinate 0. Cite this Article Format. Because the vertex appears in the standard form of the quadratic function, this form is also known as the vertex form of a quadratic function. Online Courses Study Guides Flashcards. Jennifer Ledwith is the owner of tutoring and test-preparation company Scholar Ready, LLC and a professional writer, covering math-related topics. Question 5: Which equation is represented by the graph below? How to Find the Y-Intercept of a Parabola. Rewrite the quadratic in standard form vertex form. The domain of any quadratic function as all real numbers. Parabola Changes in Quadratic Functions. In real life, this often refers to the starting point when something is being measured.

The next conic section we will look at is a parabola.

Create profiles for personalised advertising. Using this information, find the rise and run to identify the slope. The graph is also symmetric with a vertical line drawn through the vertex, called the axis of symmetry. Use limited data to select content. Did you have an idea for improving this content? Which variable represents the y-intercept for a quadratic equation in standard form:. The correct answer is D. To find the x -coordinate for the vertex we use the following formula:. Y-Intercept Overview. Finding the y-intercept of a parabola can be tricky. How To: Given a quadratic function, find the domain and range. The y-intercept has two parts: the x-value and the y-value.