Two way anova in excel 2010

Lean Six Sigma Microsoft Excel. ANOVA covers a range of common analyses. When the levels of a factor are selected at random from a wide number of possibilities, you might use a random-effects model or a mixed-effects model. And luckily, Microsoft Excel makes it easy to perform these analyses.

We use the model when we have one measurement variable and two nominal variables, also known as factors or main effects. To employ this analysis, we need to have measurements for all possible combinations of the nominal values. The method estimates how the mean of quantitative variable changes in connection to the different levels positions of two categorical values. In other words, this form of ANOVA helps analyze how to independent variables combinedly influence a dependent variable from a statistical point of view. We can also employ the method to evaluate whether the two independent factors have a significant interaction effect. To run the Two-Way ANOVA model, we need to collect data on the quantitative dependent variable at different combinations levels of two independent categorical variables.

Two way anova in excel 2010

The data set is divided into horizontal groups that are each affected by a different level of one categorical factor. The same data set is also simultaneously divided into vertical groups that are each affected by a different level of another categorical factor. An example of a data set that is arranged for two-factor ANOVA with replication analysis is as follows:. The test for main effects of each of the two factors is very similar to main effects test of the one factor in single-factor ANOVA. The main effects test for each of the two factors determines whether there is a significant difference between the means of the groups the levels within that factor. The interaction test determines whether data values across the levels of one factor vary significantly at different levels of the other factor. This test determines whether the levels of one factor have different effects on the data values across the levels of the other factor. It determines whether there is interaction between Factor 1 and Factor 2, that is, between rows and columns. Ultimately this test determines whether the differences between data observations in columns vary from row to row and the differences between data observations vary from column to column. The two factors and their levels are categorical. The dependent variable is a continuous variable. Each factor has at least two or more levels.

Because of within-group variation and bias, two way anova in excel 2010, comparisons among groups become harder. Each of the two F Tests that are main effects tests for the two factors should have their sample groups evaluated for homoscedasticity similarity of variances. The hypothesis test confirms what we might have expected from the examination of the averages: The effect of the different tapes depends on the box type.

Effect size is a way of describing how effectively the method of data grouping allows those groups to be differentiated. A simple example of a grouping method that would create easily differentiated groups versus one that does not is the following. Imagine a large random sample of height measurements of adults of the same age from a single country. If those heights were grouped according to gender, the groups would be easy to differentiate because the mean male height would be significantly different than the mean female height. If those heights were instead grouped according to the region where each person lived, the groups would be much harder to differentiate because there would not be significant difference between the means and variances of heights from different regions. Because the various measures of effect size indicate how effectively the grouping method makes the groups easy to differentiate from each other, the magnitude of effect size tells how large of a sample must be taken to achieve statistical significance. A small effect can become significant if a larger enough sample is taken.

The fact that Microsoft Excel can only handle balancing designs in which each sample does have an equal amount of observations is among its most notable restrictions. From a technical standpoint, doing a Two-Way ANOVA with an asymmetrical structure is much more complicated and challenging, and you will require some statistical package to do this. As we are aware, ANOVA is used to determine the mean difference between groups that are larger than two. ANOVA is a statistical analysis technique that divides methodical components from different variables to account for the apparent collective variation within a data set. Although there are many different types of ANOVA , the main goal of this family of studies is to ascertain if variables are associated with an outcome variable. A two-way ANOVA is performed as a statistical test to ascertain how two or more explanatory regression models would affect a continuous result variable. Whenever there is one measurement parameter and two independent parameters referred to as determinants or primary effects we employ the approach. We require observations for each conceivable variation of the theoretical amounts in order to use this methodology. But by default Excel disables this ToolPak from the ribbon. To enable this feature we need to follow the following steps.

Two way anova in excel 2010

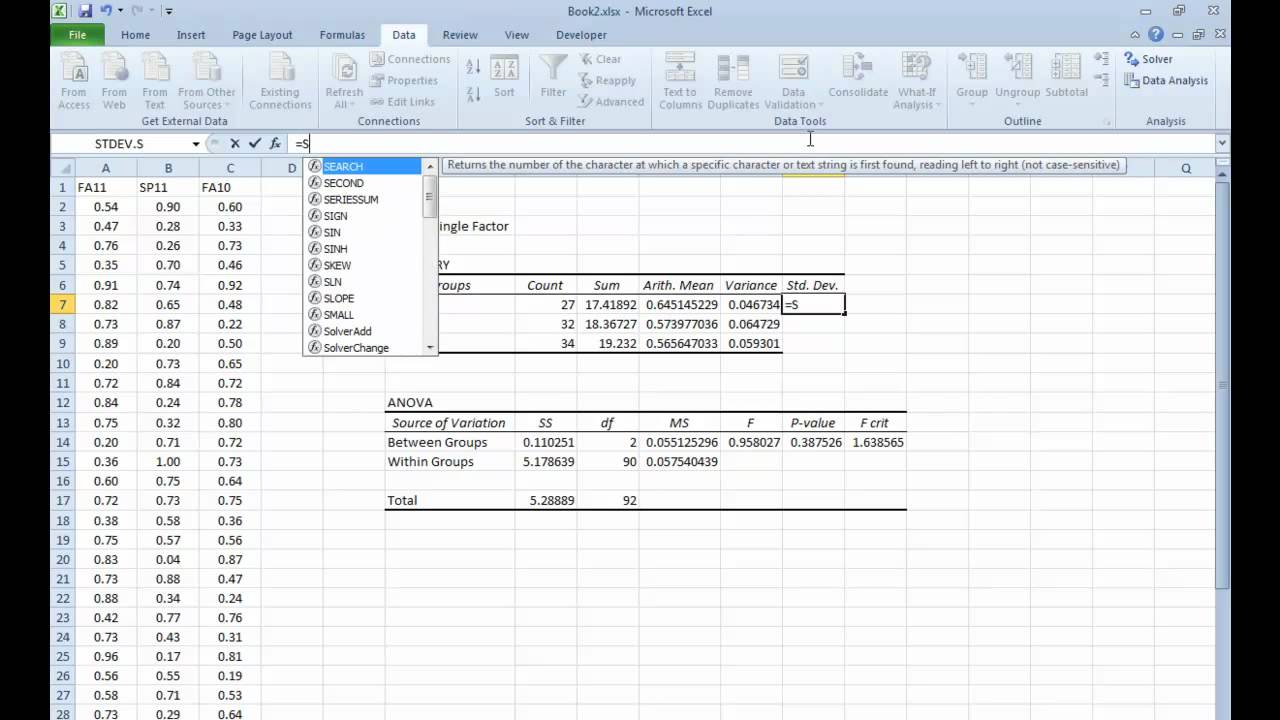
A botanist wants to know whether or not plant growth is influenced by sunlight exposure and watering frequency. She plants 40 seeds and lets them grow for two months under different conditions for sunlight exposure and watering frequency. After two months, she records the height of each plant. The results are shown below:. In the table above, we see that there were five plants grown under each combination of conditions. For example, there were five plants grown with daily watering and no sunlight and their heights after two months were 4. On the Data tab, click Data Analysis :. For example, there were multiple plants that were grown with no sunlight exposure and daily watering. The first three tables show summary statistics for each group. For example:.

Eporner.cok

The Null Hypothesis for the F Test that compares the means of the Factor 1 levels states that all of the means are the same. Factors are the variables that you will use to categorize your outcome variable into groups. Ultimately this test determines whether the differences between data observations in columns vary from row to row and the differences between data observations vary from column to column. Subscribe to my RSS Feed. As the most widely-available tool in these industries is Excel, we can find the model within the in-built Analysis Tool Pack within the software. Toggle navigation GoSkills. Each of these three F Tests produces its own p value and a result that is reported separately from the other two F Tests. Download your data files Follow along with the steps in the article by downloading these practice files Enter your email address. If the relationship is linear, eta squared will have the same value as r squared. Sample groups must be differentiated in such a way that there can be no cross-over of data between sample groups. Running a Two-Way ANOVA with an unbalanced design is significantly more complex and challenging from a computational perspective, and you will need some statistics software to perform this. Once our data is formatted in this way, and we have ensured to include an equal quantity of observations for each combination of the two categories, we can run the ANOVA model. Eta square is affected by the number and size of the other effects.

.

This F Test determining whether at least one level of the Factor 2 groupings of the data set has a significantly different mean than the other Factor 2 levels. This is a Main Effects test. The three levels of Factor 1 would, in this case, would specify which training program each person had undergone. Note that variances only have to be similar in groups of a single F Test. Small, medium, and large are relative terms. Subscribe to: Post Comments Atom. We have obtained information on the annual Salary for ten people for each group made by all combinations of factor levels. The data for the dependent variable should follow a bell curve or be normally distributed. Each categorical value should have finite possible values or factor levels. ANOVA is a parametric test that relies upon calculation of the means of sample groups. To employ this analysis, we need to have measurements for all possible combinations of the nominal values. Subscribe, and join , others. Two-Way ANOVA with one factor that has two levels and a second factor that has three levels would have a total of six unique treatment cells. Factor 1 and 2 Interaction Effects F Test Requirement If the two points above are true, then all interaction groupings the unique treatment cells will have similar variance and be normally distributed.