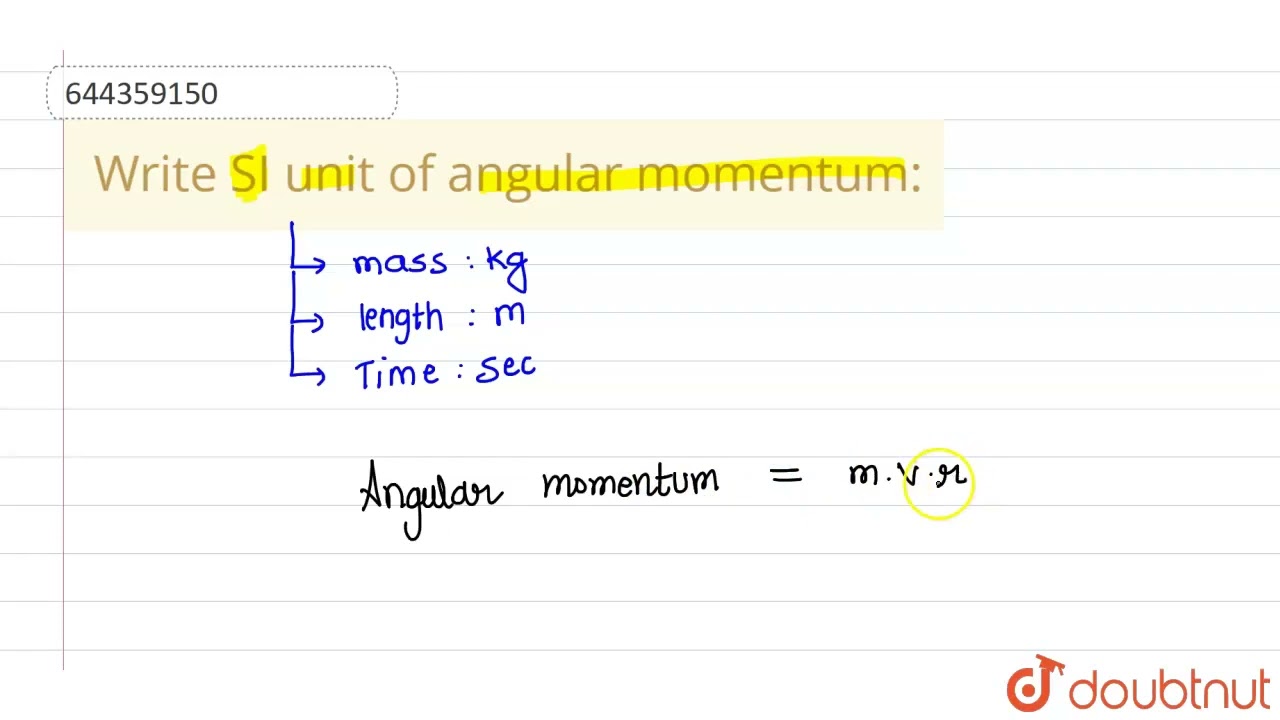
Si unit of angular momentum is

Angular Momentum is a kinematic characteristic of a system with one or more point si unit of angular momentum is. Angular momentum is sometimes called Rotational Momentum or Moment of Momentum, which is the rotational equivalent of linear momentum. It is an important physical quantity as it is conserved for a closed system and follows the Law of Conservation of Angular Momentum.

Angular momentum : The vector product of the distance r and linear momentum mv. Additional Information. Last updated on Dec 30, HTET Notificatoon to be out soon! Candidates applied online from 30th October to 11th November This is a great opportunity for teaching job aspirants.

Si unit of angular momentum is

Momentum is the product of mass and the velocity of the object. Any object moving with mass possesses momentum. The only difference in angular momentum is that it deals with rotating or spinning objects. So is it the rotational equivalent of linear momentum? If you try to get on a bicycle and balance without a kickstand, you will probably fall off. But once you start pedalling, these wheels pick up angular momentum. They are going to resist change, thereby making balancing gets easier. It is the property of a rotating body given by the product of the moment of inertia and the angular velocity of the rotating object. It is a vector quantity , which implies that the direction is also considered here along with magnitude. Point object: The object accelerating around a fixed point. For example, Earth revolving around the sun.

The unit of rotational inertia of a body in C.

In physics , angular momentum sometimes called moment of momentum or rotational momentum is the rotational analog of linear momentum. It is an important physical quantity because it is a conserved quantity — the total angular momentum of a closed system remains constant. Angular momentum has both a direction and a magnitude, and both are conserved. Bicycles and motorcycles , flying discs , [1] rifled bullets , and gyroscopes owe their useful properties to conservation of angular momentum. Conservation of angular momentum is also why hurricanes [2] form spirals and neutron stars have high rotational rates. In general, conservation limits the possible motion of a system, but it does not uniquely determine it. Unlike linear momentum, angular momentum depends on where this origin is chosen, since the particle's position is measured from it.

Momentum is the product of mass and the velocity of the object. Any object moving with mass possesses momentum. The only difference in angular momentum is that it deals with rotating or spinning objects. So is it the rotational equivalent of linear momentum? If you try to get on a bicycle and balance without a kickstand, you will probably fall off. But once you start pedalling, these wheels pick up angular momentum. They are going to resist change, thereby making balancing gets easier. It is the property of a rotating body given by the product of the moment of inertia and the angular velocity of the rotating object. It is a vector quantity , which implies that the direction is also considered here along with magnitude.

Si unit of angular momentum is

Dive into the world of rotational motion as we explore these important concepts. By understanding these principles, we can predict the behavior of rotating objects in real-world examples, such as spinning figure skaters or orbiting planets. Join us as we break down the equations, provide examples, and discuss the conservation of angular momentum. The first quantity is the angular velocity. Angular velocity describes how quickly an object is rotating and is measured in radians per second. The moment of inertia varies depending on the shape of the object. This formula can be used when studying a point mass m moving in a circular path with a linear velocity v at a distance r from the axis of rotation.

Punisher

It turns out that the best that one can do is to simultaneously measure both the angular momentum vector's magnitude and its component along one axis. Mathematics Coordinate system Differential geometry Dyadic algebra Euclidean geometry Exterior calculus Multilinear algebra Tensor algebra Tensor calculus. West Bengal Judicial Service. S2CID MH SET. CG Vyapam Stenographer. Indeed, given initial conditions of position and velocity for every point, and the forces at such a condition, one may use Newton's second law to calculate the second derivative of position, and solving for this gives full information on the development of the physical system with time. The change in angular momentum for a particular interaction is called angular impulse , sometimes twirl. SSC JE. Add Other Experiences. Login To View Results.

Angular Momentum is a kinematic characteristic of a system with one or more point masses. Angular momentum is sometimes called Rotational Momentum or Moment of Momentum, which is the rotational equivalent of linear momentum.

For other uses, see Orbital angular momentum disambiguation. Noether's theorem states that every conservation law is associated with a symmetry invariant of the underlying physics. Vizag Steel Trade Apprentice. Conservation is not always a full explanation for the dynamics of a system but is a key constraint. Rajasthan High Court Civil Judge. Patna High Court Computer Operator. Thank you for your valuable feedback! Indian Navy Tradesman. Maharashtra Agriculture Assistant. What kind of Experience do you want to share? BRO Operator Communication. If you position your right hand so that the fingers point in the direction of r. The Euler Archive. NVS Lab Attendant. Indian Army Nursing Assistant.