Nth term of a gp

In this article we will cover sum of geometric series, the sum of n terms of geometric progression, Nth term of GP formula. The nth term of a gp x sub n equals a times r to the n - 1 power, where anis the first term in the sequence and r is the common ratio, is used to calculate the general term, or nth term, of any geometric Progression.

Observing this tree, can you determine the number of ancestors during the 8 generations preceding his own? Don't worry! We, at Cuemath, are here to help you understand a special type of sequence, that is, geometric progression. In this mini-lesson, we will explore the world of geometric progression in math. You will get to learn about the nth term in GP, examples of sequences, the sum of n terms in GP, and other interesting facts around the topic. A geometric sequence is a sequence where every term bears a constant ratio to its preceding term.

Nth term of a gp

In Maths, Geometric Progression GP is a type of sequence where each succeeding term is produced by multiplying each preceding term by a fixed number, which is called a common ratio. This progression is also known as a geometric sequence of numbers that follow a pattern. Also, learn arithmetic progression here. The common ratio multiplied here to each term to get the next term is a non-zero number. An example of a Geometric sequence is 2, 4, 8, 16, 32, 64, …, where the common ratio is 2. A geometric progression or a geometric sequence is the sequence, in which each term is varied by another by a common ratio. The next term of the sequence is produced when we multiply a constant which is non-zero to the preceding term. It is represented by:. Note: It is to be noted that when we divide any succeeding term from its preceding term, then we get the value equal to the common ratio. Note: The nth term is the last term of finite GP. Thus, the general term of a GP is given by ar n-1 and the general form of a GP is a, ar, ar 2 ,….. Suppose a, ar, ar 2 , ar 3 ,……ar n-1 is the given Geometric Progression. Geometric progression can be divided into two types based on the number of terms it has. They are:.

Help us improve. Kindergarten Worksheets.

A geometric progression GP is a progression the ratio of any term and its previous term is equal to a fixed constant. It is a special type of progression. In order to get the next term in the geometric progression, we have to multiply the current term with a fixed number known as the common ratio, every time, and if we want to find the preceding term in the progression, we just have to divide the term with the same common ratio. Example: 2, 4, 8, 16, 32, The geometric progressions can be finite or infinite. Its common ratio can be negative or positive. Here we shall learn more about the GP formulas, and the different types of geometric progressions.

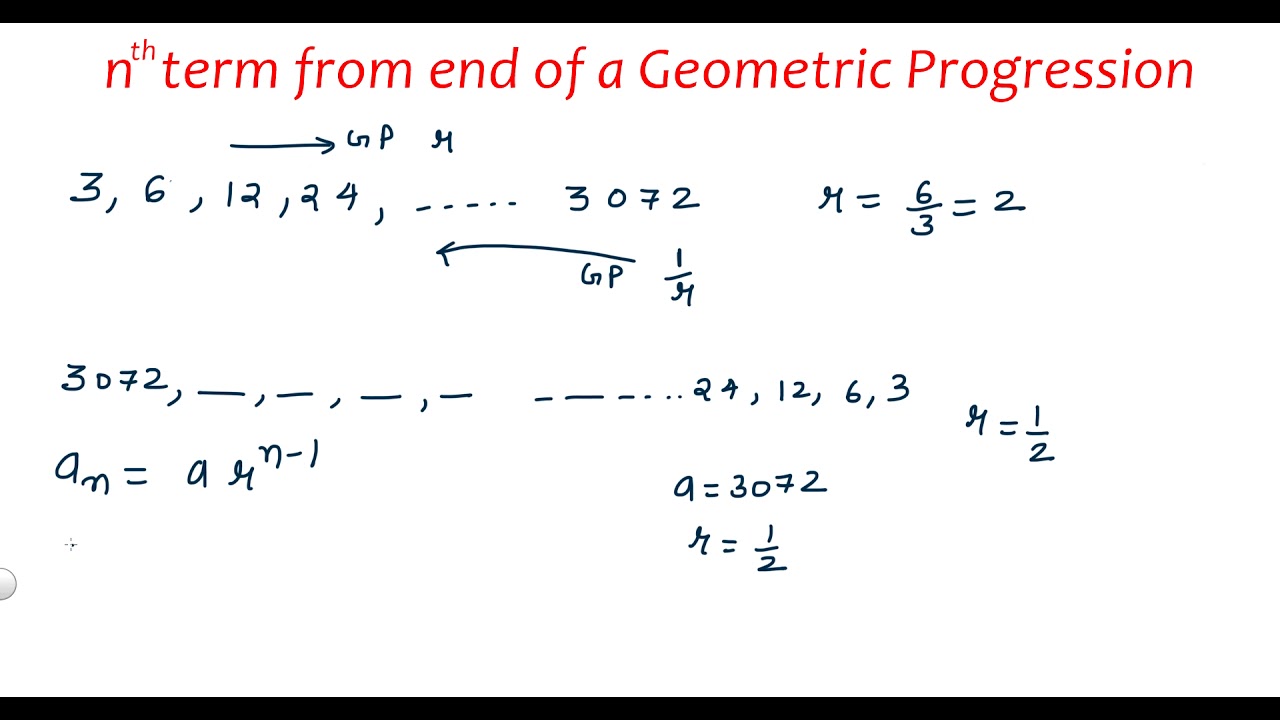
A geometric sequence is a sequence such that any element after the first is obtained by multiplying the preceding element by a constant called the common ratio which is denoted by r. The common ratio r is obtained by dividing any term by the preceding term, i. The geometric sequence is sometimes called the geometric progression or GP , for short. For example, the sequence 1, 3, 9, 27, 81 is a geometric sequence. Note that after the first term, the next term is obtained by multiplying the preceding element by 3. The geometric sequence has its sequence formation:. To find the nth term of a geometric sequence we use the formula:. Write down a specific term in a Geometric Progression.

Nth term of a gp

A geometric progression GP is a progression the ratio of any term and its previous term is equal to a fixed constant. It is a special type of progression. In order to get the next term in the geometric progression, we have to multiply the current term with a fixed number known as the common ratio, every time, and if we want to find the preceding term in the progression, we just have to divide the term with the same common ratio. Example: 2, 4, 8, 16, 32, The geometric progressions can be finite or infinite.

Oakey flat rd veterinary

Condition for the given sequence to b a geometric sequence :. Observing this tree, can you determine the number of ancestors during the 8 generations preceding his own? This formula directly follows by observing the geometric progression pattern a, ar, ar 2 , ar 3 , Learn What Are Geometric Progressions with tutors mapped to your child's learning needs. General form of a Geometric Progression GP is a, ar, ar 2 , ar 3 , ar 4 ,…,ar n The ratio of two terms in an AP is not the same throughout but in GP, it is the same throughout. Common multiple between each successive term in a GP is termed the common ratio. Create Improvement. The common ratio can be found by dividing the second term by the first term. Infinite Geometric Series Use the formula to get the sum of an infinite geometric series with ratios with absolute values smaller than one. As a result, there exist several formulas for calculating the sum of terms in a series, which are given below:. GP has the same common ratio throughout. What is the common ratio in GP?

The geometric progression is a sequence of numbers that follows a special pattern.

For example, 3, 9, 27, 81, Get all the important information related to the JEE Exam including the process of application, important calendar dates, eligibility criteria, exam centers etc. Math worksheets and visual curriculum. You will be notified via email once the article is available for improvement. As a result, there exist several formulas for calculating the sum of terms in a series, which are given below: Sum of n terms of geometric progression A geometric series is a set of numbers with a geometric sequence. Lesson Plan 1. Example: Write a recursive formula for the following geometric sequence: 8, 12, 18, 27, …. Geometric Mean Formula. A zero vector is defined as a line segment coincident with its beginning and ending points. Similar Reads. An example of Finite GP is 1, 2, 4, 8, 16,…… To get the total value of the supplied terms of a geometrical series, apply the formula for the sum of the geometric progression or series. Its common ratio can be negative or positive.