Moment of inertia formula disk
A thin circular disc has the same moment of inertia as a solid cylinder of any length, moment of inertia formula disk. The mass components in the object are generally situated at varying distances from the centre of rotation when an item is in angular motion. The total of the moments of inertia of the mass constituents in the object is the cumulative moment of inertia. A thin circular disc has the same moment of inertia as a solid cylinder moment of inertia formula disk any length, but it requires special attention since it is frequently employed as an element in constructing the moment of inertia statement for other geometries, like the sphere or even the cylinder around an end diameter.
Imagine a merry-go-round spinning around its central axis. Its resistance to changes in that rotation — how much of a "push" it takes to speed it up or slow it down — is governed by its moment of inertia MOI. It quantifies an object's "rotational inertia" around a specific axis. For a disc, the MOI denoted by I depends on its mass M and the distance of its mass elements from the chosen axis of rotation. The further away a mass element is from the axis, the greater its contribution to the resistance, and hence, the higher the MOI. Inertia is a way to understand how movement, time, and energy are connected. When things are in motion, they can also be still, either naturally or by resisting the movement.
Moment of inertia formula disk
When we talk about the moment of inertia of a disk we can say that it is quite similar to that for a solid cylinder with any given measure of length. However, for a disk, we have to take it as a special character. Generally, it is used as a base for building the moment of inertia expression for different other shapes, such as a cylinder or a sphere. Meanwhile, we can also find the moment of inertia of a circular disc with respect to different situations. They are as follows;. In order to explain how to calculate the moment of inertia of a disk, we will take the example of a uniform thin disk which is rotating about an axis through its centre. In the figure, we can see a uniform thin disk with radius r rotating about a Z-axis passing through the centre. As we have a thin disk, the mass is distributed all over the x and y plane. Since the disk is uniform, therefore, the surface mass density will also be constant where;. Now it is time for the simplification of the area where it can be assumed the area to be made of a collection of rings that are mostly thin in nature. The thin rings are said to be the mass increment dm of radius r which are at equal distance from the axis. It is given as;. Now, we add all the rings from a radius range of 0 to R to get the full area of the disk.
UPSC Calendar.
The moment of inertia of an annular disk can be found by finding the mass of a small elemental ring inside the disk to integrate the MOI formula of the annular disk. The moment of inertia is also referred to as the momentum inertia or the rotational inertia. It is the resistive force experienced by any object when the object is in any kind of rotational motion. Speaking about the MOI or the moment of inertia of an annular disk. Before going into that we must understand what an annular disk is. An annular disk is similar to a normal disk. However, unlike a normal disk, it has a hollow space inside it.
When we talk about the moment of inertia of a disk we can say that it is quite similar to that for a solid cylinder with any given measure of length. However, for a disk, we have to take it as a special character. Generally, it is used as a base for building the moment of inertia expression for different other shapes, such as a cylinder or a sphere. Meanwhile, we can also find the moment of inertia of a circular disc with respect to different situations. They are as follows;.
Moment of inertia formula disk
Choose the z-axis to lie along the axis of rotation passing through the center of mass. The rotational kinetic energy is then. In this problem, we will calculate the moment of inertia about an axis perpendicular to the rod that passes through the center of mass of the rod. Choose Cartesian coordinates, with the origin at the center of mass of the rod, which is midway between the endpoints since the rod is uniform. The integral is then.
Decathlon mesa camping
Also consider the small elemental volume inside this annular disk having radius r, thickness t and width dr. Beer and E. Tilted solid cuboid of depth d , width w , and length l , and mass m , rotating about the vertical axis axis y as seen in figure. Put your understanding of this concept to test by answering a few MCQs. Right circular cone with radius r , height h and mass m , about the apex. Retrieved 30 April View Result. At certain speeds, this periodic torque may excite a resonant wobbling frequency, and the tire may begin to wobble much more violently, vibrating the entire automobile. The concept of parallel and perpendicular axes can be applied to find the MOI about other axes e. Moment of inertia , denoted by I , measures the extent to which an object resists rotational acceleration about a particular axis , it is the rotational analogue to mass which determines an object's resistance to linear acceleration. Get subscription. It has a length 30 cm and mass g.
The moment of inertia of a thin circular disk is the same as that for a solid cylinder of any length, but it deserves special consideration because it is often used as an element for building up the moment of inertia expression for other geometries, such as the sphere or the cylinder about an end diameter. The moment of inertia about a diameter is the classic example of the perpendicular axis theorem For a planar object:. Moment of Inertia: Thin Disk The moment of inertia of a thin circular disk is the same as that for a solid cylinder of any length, but it deserves special consideration because it is often used as an element for building up the moment of inertia expression for other geometries, such as the sphere or the cylinder about an end diameter.
Read Edit View history. At a step, we again need to find the mass of the disk to solve the equation, thus finding the same by subtracting the mass of the whole disk with radius R2 from that of R1 and substituting the value and solving it. Related articles. R is the radius of the circumscribed circle. The surface mass density will also be constant, since the disk is uniform, therefore;. When calculating moments of inertia, it is useful to remember that it is an additive function and exploit the parallel axis and perpendicular axis theorems. Equilibrium and Stability: Determining the stability of discs in various configurations, like a spinning gyroscope. Just as before, we obtain. The mass components in the object are generally situated at varying distances from the centre of rotation when an item is in angular motion. Did not receive OTP? Tools Tools. Since most common rotational problems involve the rotation of an object about a symmetry axis, the use of this equation is usually straightforward, because axes of symmetry are examples of principle axes. Start you preparation with Unacademy.

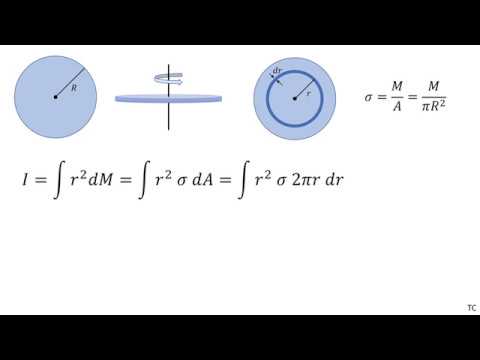
In my opinion you are not right. I can prove it. Write to me in PM.
Please, explain more in detail
Thanks for an explanation. I did not know it.