Linear approximation calculator

The idea behind local linear approximation, also called tangent line approximation or Linearizationis that we will zoom in on a point on the graph and notice that the graph now looks very similar to a line, linear approximation calculator. This means that we can use the tangent line, which rests in closeness to the curve around a point, to approximate other values along the curve as long as we stay near the particular point. But in all honesty, this linear approximation calculator nothing more than the equation of the tangent line we used in our previous lesson:.

The calculator will find the linear approximation to the explicit, polar, parametric, and implicit curve at the given point, with steps shown. Related calculator: Quadratic Approximation Calculator. The Linear Approximation Calculator uses a linear function to approximate the value of a more complex function. It allows you to calculate linear approximations of explicit, parametric, polar, or implicit curves at a given point. Begin by choosing the type of function you have, whether it's explicit, parametric, polar, or implicit. Input the function you want to approximate.

Linear approximation calculator

Instructions: Use this calculator to compute the linear approximation for a given function at a given point you provide, showing all the steps. Please type in the function and the point in the form box below. This linearization calculator will allow to compute the linear approximation, also known as tangent line for any given valid function, at a given valid point. Once you provide a valid function and point, you click on "Calculate" and all the calculations will be shown for you. The formal mathematical definition of 'barely touching' is given by the idea of tangent line , for which we need to compute the derivative of the function. As you have probably suspected by now, the linear approximation is the same as the tangent line at the given point. Then, calculating the linear approximation is exactly the same as calculating the tangent line. Another name for the same is first order approximation, or tangent line approximation, which are commonly used names in Calculus as well. Another common concept is that of differential, which is tightly linked to that of the linear approximation, and it is simply a derivation of it. So then, based on the first order approximation formula, the formula for the differential is.

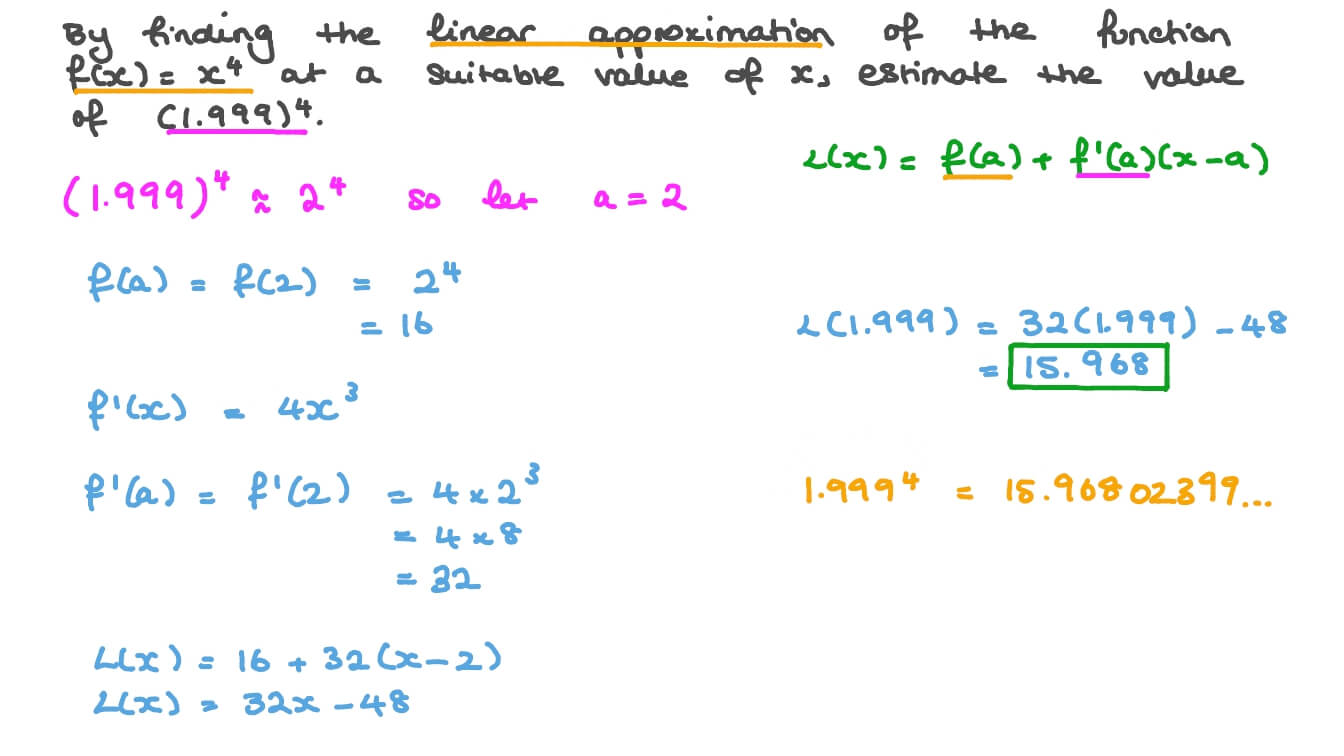
Learn math Krista King September 4, mathlearn onlineonline linear approximation calculatoronline mathcalculus 1calculus icalc 1calc ilinear approximationlinear approximation calculator, linearizationderivativesdifferentiation. So, together we will look at the overall process and idea behind linear approximation i. In fact, when we are asked to use differentials to estimate a given number, such as the square root or exponential, we are, in essence, being asked to use linearization to approximate the value.

Linear approximation is also known as a tangent line or tangent in geometry means a line or plane that intersects a curve or a curved surface at exactly one point. Online Linear Approximation Calculator helps you to calculate the value of linear approximation for a given function in a few seconds. Linear approximation is defined as the equation of a tangent line. Thus, the linear approximation formula is an application of derivatives. Similarly, you can try the linear approximation calculator.

Linear approximation is the process of simplifying a relatively complex function into a linear function that allows us to approximate f x when our x value is sufficiently close to a given value x 0. As we zoom in on the point x 0 , we can see that the curvature of f x begins flattening out and looks more like the tangent line red line. This visualization helps us understand why approximations of f x are more accurate near x 0. If we keep zooming in on the graph, f x will eventually look like a straight line. If a higher degree of accuracy is desired, using higher order polynomials via polynomial approximation will generally yield better results when compared to linear approximation. Linear approximation is more than just another topic in a mathematics class. It actually has many practical uses in the real world. One example would be the use of linear approximation to estimate the number of sales a business will make at a point in the near future.

Linear approximation calculator

This calculator can derive linear approximation formula for the given function, and you can use this formula to compute approximate values. You can use linear approximation if your function is differentiable at the point of approximation more theory can be found below the calculator. Taylor's theorem gives an approximation of a k-times differentiable function around a given point by a k-th order Taylor polynomial. Thus, by dropping the remainder h1 , you can approximate some general function using a linear function, the resulting graph is the tangent line to the graph of a general function at the point of approximation a. This is a good approximation for x when it is close enough to a , since a closely observed curve resembles a straight line. But, of course, Taylor's theorem also ensures that the quadratic approximation and other higher degree approximations is, in a sufficiently small neighborhood of the point a , a better approximation than the linear approximation. Linear approximation.

Commander decklist

Our Mission. Take a Tour and find out how a membership can take the struggle out of learning math. Finally, simplifying. We'll assume you're ok with this, but you can opt-out if you wish. How does the Linear Approximation Calculator work? To put it plainly, consider some function and the curve it represents. So then, we first need the derivative. So, together we will look at the overall process and idea behind linear approximation i. Result The calculator will provide the linear approximation of the function at the specified point. Linear Approximation Example. It allows you to calculate linear approximations of explicit, parametric, polar, or implicit curves at a given point. Although this is a simple example, it emphasizes the power of linear approximation. What types of functions can I use with the calculator?

Instructions: Use this calculator to compute the linear approximation for a given function at a given point you provide, showing all the steps.

Calculation After you have filled in all the fields, click the "Calculate" button. Expressed as a percentage of??? Fraction Calculator. So, together we will look at the overall process and idea behind linear approximation i. Then, calculating the linear approximation is exactly the same as calculating the tangent line Another name for the same is first order approximation, or tangent line approximation, which are commonly used names in Calculus as well. Online Tutors. The Linear Approximation equation Linear approximation is a useful tool because it allows us to estimate values on a curved graph difficult to calculate , using values on a line easy to calculate that happens to be close by. Take a Tour and find out how a membership can take the struggle out of learning math. Our calculator is versatile and can handle various functions, including explicit, parametric, polar, and implicit curves. Using linear approximation to estimate a function's value.