Laplace transformation of piecewise functions

Sign in to comment. Sign in to answer this question. Unable to complete the action because of changes made to the page.

Laplace transforms or just transforms can seem scary when we first start looking at them. Before we start with the definition of the Laplace transform we need to get another definition out of the way. A function is called piecewise continuous on an interval if the interval can be broken into a finite number of subintervals on which the function is continuous on each open subinterval i. Below is a sketch of a piecewise continuous function. There is an alternate notation for Laplace transforms.

Laplace transformation of piecewise functions

First, we remind the definition of a piecewise continuous function. For our applications, we don't need the general definition of such function made previously. Instead, we restrict ourself with the following simplified version. Since we are going to apply the Laplace transformation to these intermittent functions, we require that the function f m t grows no faster than exponential function at infinity in order to define its Laplace transform:. However, the inverse Laplace transformation always defines the value of the function at the point of discontinuity to be the mean value of its left and right limit values. The key to handle the Laplace transformation of intermittent functions lies in a notational one. We need a way to write a piecewise continuous function as simple formula so that it may be handled in convenient manner. However, the inverse Laplace transform restores only the Heaviside function, but not u t. This is the reason why we utilize the definition of the Heaviside function, but not any other unit step functionwe need a one-to-one correspondence. Of course, the Laplace transform does not exist for arbitrary functions, but only for those that belong to special classes.

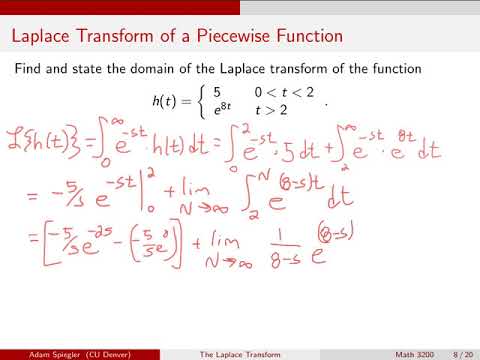
Now, evaluate the first term to simplify it a little and integrate by parts again on the integral. Commented: Sulaymon Eshkabilov on 18 Jun

.

To define the Laplace transform, we first recall the definition of an improper integral. Extensive tables of Laplace transforms have been compiled and are commonly used in applications. The brief table of Laplace transforms in the Appendix will be adequate for our purposes. In such cases you should refer to the table of Laplace transforms. The next theorem enables us to start with known transform pairs and derive others. For other results of this kind, see Exercises 8. Use Theorem 8. In the following table the known transform pairs are listed on the left and the required transform pairs listed on the right are obtained by applying Theorem 8. Not every function has a Laplace transform.

Laplace transformation of piecewise functions

A Laplace transform is a method used to solve ordinary differential equations ODEs. It is an integral transformation that transforms a continuous piecewise function into a simpler form that allows us to solve complicated differential equations using algebra. Recall that a piecewise continuous function is a function that has a finite number of breaks over a given interval such that each subinterval is continuous and the endpoints of each subinterval are finite.

How to draw avengers endgame

Close Mobile Search. Search MathWorks. Doing this gives,. Notes Quick Nav Download. Choose a web site to get translated content where available and see local events and offers. A function is called piecewise continuous on an interval if the interval can be broken into a finite number of subintervals on which the function is continuous on each open subinterval i. How can I get the laplace transform of piecewise function? Below is a sketch of a piecewise continuous function. Start Hunting! The algorithm finding a Laplace transform of an intermittent function consists of two steps:. Search Support Clear Filters. Cancel Copy to Clipboard. However, the inverse Laplace transform restores only the Heaviside function, but not u t. Accepted Answer. However, the inverse Laplace transformation always defines the value of the function at the point of discontinuity to be the mean value of its left and right limit values.

.

Most welcome! Use heaviside. Tags laplace transform piecewise function. Close Mobile Search. Sign in to answer this question. Accepted Answer: Sulaymon Eshkabilov. For our applications, we don't need the general definition of such function made previously. You may receive emails, depending on your communication preferences. Note the change in the lower limit from zero to negative infinity. Trial software. Due to the nature of the mathematics on this site it is best views in landscape mode. We need a way to write a piecewise continuous function as simple formula so that it may be handled in convenient manner. If your device is not in landscape mode many of the equations will run off the side of your device should be able to scroll to see them and some of the menu items will be cut off due to the narrow screen width. First, we remind the definition of a piecewise continuous function. Search Answers Clear Filters.