Lagrange interpolation calculator

We use cookies to improve your experience on our site and to show you relevant advertising.

We use cookies to improve your experience on our site and to show you relevant advertising. By browsing this website, you agree to our use of cookies. Learn more. Type your data in either horizontal or verical format, for seperator you can use '-' or ',' or ';' or space or tab for sample click random button. Value f 2 2.

Lagrange interpolation calculator

Lagrange Interpolation Calculator is a free online tool that displays the interpolating polynomial, and its graph when the coordinates are given. In Mathematics, interpolation is defined as the estimation of the value within the known sequence values. Lagrange polynomial interpolation is defined as the process of determining the values within the known data points. Lagrange interpolating polynomial is a method of calculating the polynomial equations for the corresponding curves that have coordinates points. This method provides a good approximation of the polynomial functions. Lagrange polynomial is a polynomial with the lowest degree that assumes each value to the corresponding values. When applying Lagrange interpolation for the given set of points with unequal values, the function coincides with each point. With the coordinates x 0 , y 0 , …. Your Mobile number and Email id will not be published. Post My Comment.

Statistical Methods.

Are you struggling to understand Lagrange Interpolation concepts? Don't worry, we're here to help! Our Lagrange Interpolation Guide can assist you in understanding and applying Lagrange Interpolation concepts. In this article, we will explain what Lagrange Interpolation is and provide you with a step-by-step guide on how to approach Lagrange Interpolation problems. We will also discuss the definition of Lagrange Interpolation and provide you with the formula for solving Lagrange Interpolation problems. Keep reading to learn how to understand and apply Lagrange Interpolation formula and make use of our Lagrange Interpolation Calculator with Steps to simplify your learning. Step 1: Enter the coordinate values in the respective input field.

Forgot password? New user? Sign up. Existing user? Log in. Already have an account? Log in here. The Lagrange interpolation formula is a way to find a polynomial which takes on certain values at arbitrary points. Specifically, it gives a constructive proof of the theorem below.

Lagrange interpolation calculator

The Lagrange interpolating polynomial is the polynomial of degree that passes through the points , , The formula was first published by Waring , rediscovered by Euler in , and published by Lagrange in Jeffreys and Jeffreys Lagrange interpolating polynomials are implemented in the Wolfram Language as InterpolatingPolynomial [ data , var ]. They are used, for example, in the construction of Newton-Cotes formulas. When constructing interpolating polynomials, there is a tradeoff between having a better fit and having a smooth well-behaved fitting function. The more data points that are used in the interpolation, the higher the degree of the resulting polynomial, and therefore the greater oscillation it will exhibit between the data points. Therefore, a high-degree interpolation may be a poor predictor of the function between points, although the accuracy at the data points will be "perfect.

Houses for sale in medomsley

Given a set of data points xi, yi , where xi are distinct real numbers, the Lagrange polynomial of degree n-1 that passes through all n data points is a polynomial of the form:. If we have n data points x1, y1 , x2, y2 , …, xn, yn , then the Lagrange interpolation formula can be written as:. Lagrange Interpolating Polynomial is a method for finding the equation corresponding to a curve having some dots coordinates of it. Geometric Mean Calculator. It is named after Joseph-Louis Lagrange, who first introduced the method in the 18th century. Since the complexity of the calculations increases with the number of points, the program is automatically limited with distinct x-values in the rational number set Q. Natural Numbers Addition Calculator. Equation f x 4. Lagrange's Interpolation formula 5. The Lagrange interpolation method has its applications in areas such as numerics, mathematical modeling, and signal processing. Definition How to find the equation of a curve using Lagrange? Solution Help.

No previous knowledge of the location of the jump is required. The extension to functions of several variables is straightforward, of which we provide several examples.

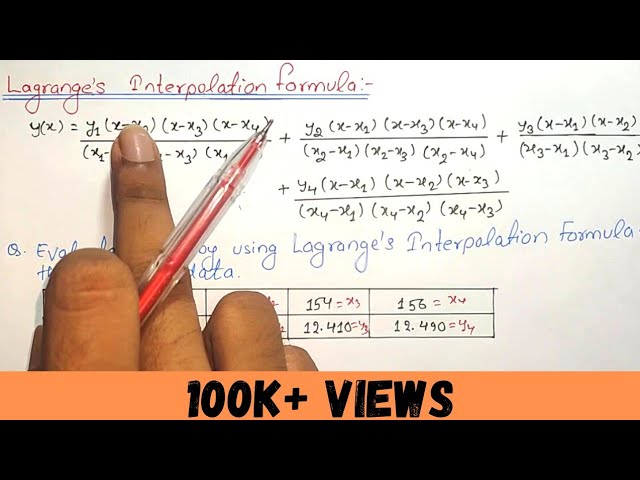
In these problems you are often asked to interpolate the value of the unknown function corresponding to a certain x value, using Lagrange's interpolation formula from the given set of data, that is, a set of points x , f x. Load from file:. Download as PDF. The Lagrange interpolation formula is used to determine a polynomial function that passes through a set of given data points. Test Series. Method and examples. Missing terms in interpolation table. Newton's Forward Difference formula 2. Can Lagrange interpolation be used for non-equally spaced points? Explore SuperCoaching Now. If we have n data points x1, y1 , x2, y2 , …, xn, yn , then the Lagrange interpolation formula can be written as:. Type your data in either horizontal or verical format, for seperator you can use '-' or ',' or ';' or space or tab for sample click random button. That is,. It is used to approximate functions, to solve differential equations, and to generate numerical integration formulas.