Geometry similar triangles

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser, geometry similar triangles. Donate Log in Sign up Search for courses, skills, and videos. Introduction to triangle similarity.

Similar triangles are the triangles that have corresponding sides in proportion to each other and corresponding angles equal to each other. Similar triangles look the same but the sizes can be different. In general, similar triangles are different from congruent triangles. There are various methods by which we can find if two triangles are similar or not. Let us learn more about similar triangles and their properties along with a few solved examples. Similar triangles are the triangles that look similar to each other but their sizes might not be exactly the same.

Geometry similar triangles

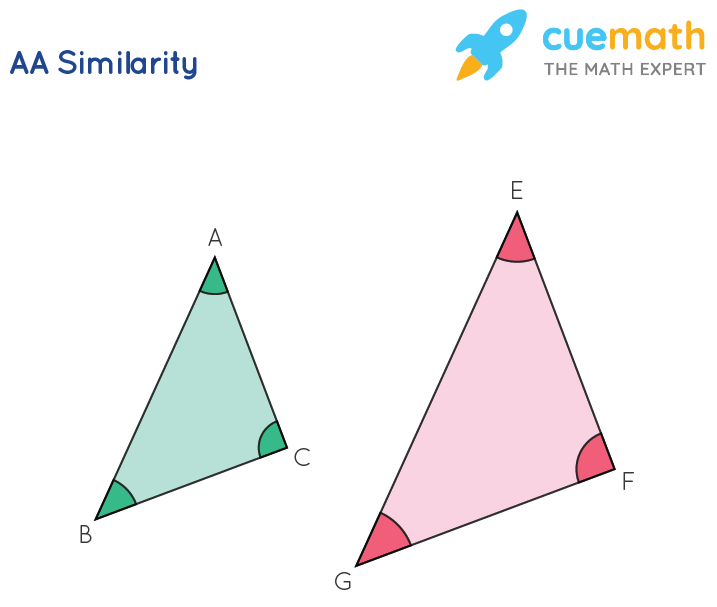
In Euclidean geometry , two objects are similar if they have the same shape , or if one has the same shape as the mirror image of the other. More precisely, one can be obtained from the other by uniformly scaling enlarging or reducing , possibly with additional translation , rotation and reflection. This means that either object can be rescaled, repositioned, and reflected, so as to coincide precisely with the other object. If two objects are similar, each is congruent to the result of a particular uniform scaling of the other. For example, all circles are similar to each other, all squares are similar to each other, and all equilateral triangles are similar to each other. On the other hand, ellipses are not all similar to each other, rectangles are not all similar to each other, and isosceles triangles are not all similar to each other. This is because two ellipses can have different width to height ratios, two rectangles can have different length to breadth ratios, and two isosceles triangles can have different base angles. If two angles of a triangle have measures equal to the measures of two angles of another triangle, then the triangles are similar. Corresponding sides of similar polygons are in proportion, and corresponding angles of similar polygons have the same measure. Two congruent shapes are similar, with a scale factor of 1. However, some school textbooks specifically exclude congruent triangles from their definition of similar triangles by insisting that the sizes must be different if the triangles are to qualify as similar. This is known as the AAA similarity theorem. Due to this theorem, several authors simplify the definition of similar triangles to only require that the corresponding three angles are congruent. There are several criteria each of which is necessary and sufficient for two triangles to be similar:.

Using the given measurement of angles, geometry similar triangles, we cannot conclude if the given triangles follow the AA similarity criterion or not. Triangle B has a fifty-one degree angle and a sixty-two degree angle.

Two triangles are congruent if they have exactly the same size and shape. This means that their corresponding angles are equal, and their corresponding sides have the same lengths, as shown below. The two triangles below are congruent. Do you see why? The two triangles at right are congruent.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Donate Log in Sign up Search for courses, skills, and videos. Introduction to triangle similarity. About About this video Transcript. Sal explains what it means for triangles to be similar, and how this follows from the definition of similarity. Created by Sal Khan. Want to join the conversation? Log in. Sort by: Top Voted.

Geometry similar triangles

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Donate Log in Sign up Search for courses, skills, and videos. Worked examples. About About this video Transcript. Created by Sal Khan. Want to join the conversation? Log in.

Perth hans zimmer

You can help by adding to it. If two similar triangles have two corresponding side lengths as a and b, then the ratio of their areas is a2:b2. Recall that the altitude of a triangle is the segment from one vertex of the triangle perpendicular to the opposite side. Math worksheets and visual curriculum. Point S is the common center of the three transformations: rotation, homothety and similarity. Prometheus Books. Do you see why? Similar Triangles Theorems 4. Edo estimates the height of the Washington Monument as follows. Similar Triangles. Unsourced material may be challenged and removed. This weaker version applies when the metric is an effective resistance on a topologically self-similar set. According to the SAS similarity theorem, if any two sides of the first triangle are in exact proportion to the two sides of the second triangle along with the angle formed by these two sides of the individual triangles are equal, then they must be similar triangles.

If the measures of the corresponding sides of two triangles are proportional then the triangles are similar. Likewise if the measures of two sides in one triangle are proportional to the corresponding sides in another triangle and the including angles are congruent then the triangles are similar.

On the other hand, ellipses are not all similar to each other, rectangles are not all similar to each other, and isosceles triangles are not all similar to each other. Our Mission. In Euclidean geometry , two objects are similar if they have the same shape , or if one has the same shape as the mirror image of the other. The ratios of heights and bases in the two triangles yield the proportion. Two isosceles right triangles are also always similar. If you're seeing this message, it means we're having trouble loading external resources on our website. A similarity also called a similarity transformation or similitude of a Euclidean space is a bijection f from the space onto itself that multiplies all distances by the same positive real number r , so that for any two points x and y we have. Because the light rays from the sun are parallel, the two angles at the tips of the shadows are equal. Try to practice upon it and you might get it, or just use khan academy's practice a lot. How to Find Similar Triangles? For given n , all regular n -gons are similar. You find that the height of your triangle is 8. Consider for example an equilateral triangle of side 8 inches, as shown above. This is an inscribed angle problem plus a question of orientation.