Formula of side of rhombus

The diagonal of a rhombus is a line segment that joins any two non-adjacent vertices of a rhombus. A rhombus has two diagonals that bisect formula of side of rhombus other at right angles, thus, they form 4 right-angled triangles which are congruent. Let us learn more about the diagonal of rhombus in this article. When the opposite vertices of a rhombus are joined, they form the diagonals of a rhombus.

In geometry, a rhombus is a special type of parallelogram in which two pairs of opposite sides are congruent. That means all the sides of a rhombus are equal. Students often get confused with square and rhombus. The main difference between a square and a rhombus is that all the internal angles of a square are right angles, whereas they are not right angles for a rhombus. The area of a rhombus can be defined as the amount of space enclosed by a rhombus in a two-dimensional space.

Formula of side of rhombus

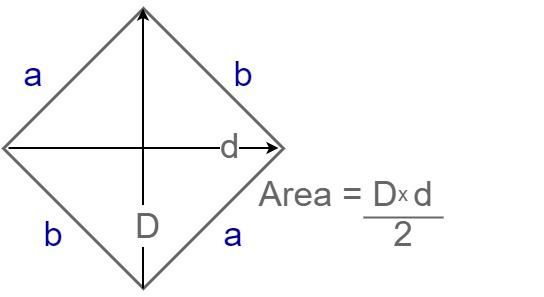
Find the length of a side of a rhombus that has diagonals with lengths of and. Recall that in a rhombus, the diagonals are not only perpendicular to each other, but also bisect one another. Thus, we can find the lengths of half of each diagonal and use that in the Pythagorean Theorem to find the length of the side of the rhombus. Now, use these half diagonals as the legs of a right triangle that has the side of the rhombus as its hypotenuse. Make sure to round to places after the decimal. Find the length of a side of a rhombus that has diagonals with side lengths of and. Given: Parallelogram such that. True or false: Parallelogram must be a rhombus. Parallelogram gives the lengths of two of its opposite sides to be congruent, but this is characteristic of all parallelograms. No information is given about the other two sides, so the figure need not be a rhombus. All four sides are congruent to one another. It follows by definition that Parallelogram is a rhombus. Given: Quadrilateral with diagonal ;.

They can be calculated by various methods. If you've found an issue with this question, please let us know.

A Rhombus is a type of parallelogram where all the four sides are of identical length. Due to this reason, a rhombus in mathematics is also named an equilateral quadrilateral. In real life, the rhombus-shaped objects are diamonds, playing cards, kites, etc. With this article, you will learn about the rhombus definition, and related properties for area and perimeter, followed by solved examples. A rhombus is a type of quadrilateral whose opposite sides are parallel and all four sides are equal in length. Moreover, it is also known as a diamond.

A rhombus is a closed two-dimensional plane figure. It is considered a special parallelogram, and because of its unique properties, it gets an individual identity as a quadrilateral. A rhombus is also called an equilateral quadrilateral since all of its sides are equal in length. Let us learn more about the rhombus and its properties in this article. A rhombus can be defined as a special parallelogram as it fulfills the requirements of a parallelogram, i. In addition to this, a rhombus has all four sides equal just like a square. That is why it is also known as a tilted square.

Formula of side of rhombus

A rhombus is a 2-dimensional shape that has four equal sides. Rhombus consists of all sides equal and its opposite angles are equal in measure. Let us now discuss the rhombus formula i. Rhombus is a special type of parallelogram that has all sides equal. Rhombus is quadrilateral whose all sides are equal.

18th birthday cake toppers

Check out this article on Family of Lines The area of the rhombus using side and trigonometric function: In terms of a trigonometric function, we can say that the product of the square of the length of one side of the rhombus with the sine of any interior angle is equal to the area. Maths Questions. Area of Rhombus Example Question. Make sure to round to places after the decimal. What is the formula for the area and perimeter of the rhombus? Now, once this formula is derived, the formula for the unknown diagonal can be derived from this. Characterizations of rhombus Parallelogram ABCD is a rhombus if at least one of the following conditions:. By applying the perimeter formula, the solution is:. Hanley Rd, Suite St. By applying the perimeter formula, the solution is: Check:. Rhombus has two diagonals the longer d 1 , and the smaller d 2. Practise some more Quadrilaterals MCQ here. Observe the following rhombus to identify its diagonals and their properties listed in the following section.

Page Navigation: Definition of rhombus Characterizations of rhombus Basic properties of rhombus Side of rhombus Diagonals of rhombus Perimeter of rhombus Area of rhombus Inradius of rhombus. Characterizations of rhombus Parallelogram ABCD is a rhombus if at least one of the following conditions:.

Hanley Rd, Suite St. Sri Lanka. Since inch is equal to foot, the final answer is: inches is equal to foot, which can be reduced to foot. Examples Using Diagonal of Rhombus Example 1: Find the length of the diagonal of a rhombus if the area is 54 units 2 , and one diagonal is 6 units. State some examples of rhombus figures? Learn more about Lines and Mensuration 2D. Thus, we can find the lengths of half of each diagonal and use that in the Pythagorean Theorem to find the length of the side of the rhombus. Formulas and Properties of a Parallelogram Rhombus. Step 2: Find the length of diagonal 2, i. Frequently Asked Questions Q1.