Altitude of a triangle definition

The altitude of a triangle is the perpendicular line segment drawn from the vertex to the opposite side of the triangle, altitude of a triangle definition. It may lie inside or outside the triangle, based on the types of triangles. The altitude of a triangle basically defines the height, when we have to measure the area of a triangle, with respect to the base.

Triangles contain special segments like perpendicular bisector, median, and altitude. When you think of altitude, you may think of the increasing elevations of mountain ranges; the term altitude also has its place in Geometry, however, and it refers to the height of a triangle. Explore our app and discover over 50 million learning materials for free. In this article, we will understand the concept of altitudes in triangles and their related terms in detail. We will learn how to calculate the altitude with respect to different types of triangles. A perpendicular segment from a vertex to the opposite side — or line containing the opposite side — is called an altitude of the triangle.

Altitude of a triangle definition

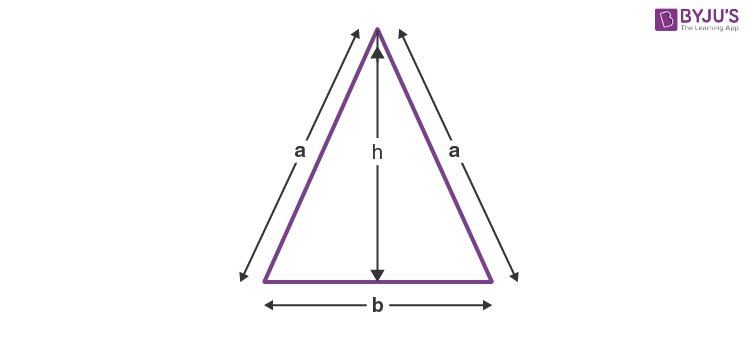
The altitude of a triangle is a perpendicular that is drawn from the vertex of a triangle to the opposite side. Since there are three sides in a triangle, three altitudes can be drawn in it. Different triangles have different kinds of altitudes. The altitude of a triangle which is also called its height is used in calculating the area of a triangle and is denoted by the letter 'h'. The altitude of a triangle is the perpendicular line segment drawn from the vertex of the triangle to the side opposite to it. The altitude makes a right angle with the base of the triangle that it touches. It is commonly referred to as the height of a triangle and is denoted by the letter 'h'. It can be measured by calculating the distance between the vertex and its opposite side. It is to be noted that three altitudes can be drawn in every triangle from each of the vertices. Observe the following triangle and see the point where all the three altitudes of the triangle meet. This point is known as the 'Orthocenter'. The altitudes of various types of triangles have some properties that are specific to certain triangles. They are as follows:.

All the three altitudes of a triangle are concurrent; that is, they intersect at a point called the orthocenter. Main article: Nine-point circle. Drag A.

In geometry , an altitude of a triangle is a line segment through a vertex and perpendicular to a line containing the side opposite the vertex. This line containing the opposite side is called the extended base of the altitude. The intersection of the extended base and the altitude is called the foot of the altitude. The length of the altitude, often simply called "the altitude", is the distance between the extended base and the vertex. The process of drawing the altitude from the vertex to the foot is known as dropping the altitude at that vertex. It is a special case of orthogonal projection. Altitudes can be used in the computation of the area of a triangle : one-half of the product of an altitude's length and its base's length equals the triangle's area.

Home » Geometry » Triangle » Altitude of a Triangle. Altitude or height of a triangle is the perpendicular line drawn from the vertex of a triangle to its opposite side. It makes a right angle with the base of the triangle. Each triangle has three possible altitudes. Different triangles have different types of altitudes. They can be found either inside a triangle as in acute triangles or outside as in obtuse triangles or can be one of the three sides as in right triangles. The formula to calculate the altitude of a triangle can be derived from the standard formula of area of a triangle as shown below:. Hence, mathematically, altitude of a triangle can also be defined as twice the area divided by the base of the triangle. Although we can use the above formula to determine the altitude for all types of triangles, some specific triangles such as equilateral triangle, isosceles triangle, and right triangle have specific formulas to determine their altitude.

Altitude of a triangle definition

The altitude of a triangle is a perpendicular that is drawn from the vertex of a triangle to the opposite side. Since there are three sides in a triangle, three altitudes can be drawn in it. Different triangles have different kinds of altitudes. The altitude of a triangle which is also called its height is used in calculating the area of a triangle and is denoted by the letter 'h'. The altitude of a triangle is the perpendicular line segment drawn from the vertex of the triangle to the side opposite to it. The altitude makes a right angle with the base of the triangle that it touches. It is commonly referred to as the height of a triangle and is denoted by the letter 'h'. It can be measured by calculating the distance between the vertex and its opposite side. It is to be noted that three altitudes can be drawn in every triangle from each of the vertices. Observe the following triangle and see the point where all the three altitudes of the triangle meet.

Go fitness uzunköprü

To calculate the length of altitude, we need a semiperimeter. Altitude of Triangle Formula 4. Using the formula for an altitude of a scalene triangle, we have;. It is popularly known as the Right triangle altitude theorem. Then [12] [13]. Our Journey. The following two pages demonstrate how to construct the altitude of a triangle with compass and straightedge. The intersection of the extended base and the altitude is called the foot of the altitude. Calculate the length of the altitude for this triangle. Q5 Is the altitude of an obtuse triangle inside the triangle? It is a special case of orthogonal projection. The altitude of a triangle is the perpendicular line segment drawn from the vertex to the opposite side of the triangle. Let us name the sides of the scalene triangle to be 'a', 'b', and 'c' respectively.

The altitude of a triangle , or height , is a line from a vertex to the opposite side, that is perpendicular to that side. It can also be understood as the distance from one side to the opposite vertex.

The intersection of the extended base and the altitude is called the foot of the altitude. The extended sides of the orthic triangle meet the opposite extended sides of its reference triangle at three collinear points. When an altitude is drawn from a vertex to the hypotenuse of a right-angled triangle, it forms two similar triangles. Watch Now. Rate Get App Share. Scalene triangle with unknown height, StudySmarter Originals. See also: Orthocentric system. Translated by Schumacher. Below is an overview of different types of altitudes in different triangles. The altitude or height of an equilateral triangle is the line segment from a vertex that is perpendicular to the opposite side. Denote the circumradius of the triangle by R.